Imagine you’re navigating a vast ocean, with a compass guiding your way. But this isn’t just any compass; it’s a magical tool that unveils the hidden relationships between angles and the coordinates of points on a circle. This is the essence of the Unit Circle, a fundamental tool in trigonometry that reveals the values of sine, cosine, and tangent for any angle between 0 and 360 degrees. Like a secret map, the Unit Circle can unlock a world of possibilities in mathematics, science, and engineering, empowering you to solve complex problems and understand the intricate world around us.

Image: trudiogmor.blogspot.com
This journey into the Unit Circle might seem daunting at first, but fear not, for it is a voyage of discovery. We will unravel its mysteries step-by-step, exploring the historical context that birthed this powerful tool, decoding its core principles, and ultimately showing you how to master it. Just like a skilled navigator uses a compass to find their bearings, you can become a master of trigonometric values equipped with the knowledge and tools provided in this article.
Embarking on a Journey through the Unit Circle:
The Unit Circle is a magical circle with a radius of 1, centered at the origin of a coordinate plane. Its magic lies in its ability to represent every angle between 0 and 360 degrees using points on its circumference. Each point on this circle corresponds to a specific angle, and its coordinates reveal the values for sine, cosine, and tangent of that angle.
Imagine a line, like a ray of sunlight, emanating from the origin and rotating counter-clockwise. This rotating line defines the angle, measured in degrees or radians. As this line sweeps around the circle, it intersects the circumference at different points, each representing unique trigonometric values.
Let’s break down the key concepts:
- Angles: The angle is the measure of the rotation of the ray from the initial position, which is usually the positive x-axis, to its final position.
- Sine: The sine of an angle is the y-coordinate of the point where the rotating ray intersects the Unit Circle.
- Cosine: The cosine of an angle is the x-coordinate of the point where the rotating ray intersects the Unit Circle.
- Tangent: The tangent of an angle is the ratio of sine to cosine, which can be visualized as the slope of the line connecting the origin and the point on the Unit Circle.
Navigating the Quadrants:
The Unit Circle is divided into four quadrants, each representing a different range of angles.
- Quadrant I (0° to 90°): All trigonometric functions (sine, cosine, tangent) are positive in this quadrant.
- Quadrant II (90° to 180°): Only sine is positive, while cosine and tangent are negative.
- Quadrant III (180° to 270°): Only tangent is positive, while sine and cosine are negative.
- Quadrant IV (270° to 360°): Only cosine is positive, while sine and tangent are negative.
Understanding this quadrantal relationship is crucial for quickly determining the signs of trigonometric functions for any given angle.
Unveiling the Key Angles:
Now, let’s look at the specific values of sine, cosine, and tangent for certain key angles within the Unit Circle. These angles are considered fundamental and are frequently used in various mathematical and scientific applications.
Common Angles (in degrees):
| Angle | Sine | Cosine | Tangent |
|---|---|---|---|
| 0° | 0 | 1 | 0 |
| 30° | 1/2 | √3/2 | 1/√3 |
| 45° | √2/2 | √2/2 | 1 |
| 60° | √3/2 | 1/2 | √3 |
| 90° | 1 | 0 | Undefined |
| 120° | √3/2 | -1/2 | -√3 |
| 135° | √2/2 | -√2/2 | -1 |
| 150° | 1/2 | -√3/2 | -1/√3 |
| 180° | 0 | -1 | 0 |
| 210° | -1/2 | -√3/2 | 1/√3 |
| 225° | -√2/2 | -√2/2 | 1 |
| 240° | -√3/2 | -1/2 | √3 |
| 270° | -1 | 0 | Undefined |
| 300° | -√3/2 | 1/2 | -√3 |
| 315° | -√2/2 | √2/2 | -1 |
| 330° | -1/2 | √3/2 | -1/√3 |
| 360° | 0 | 1 | 0 |
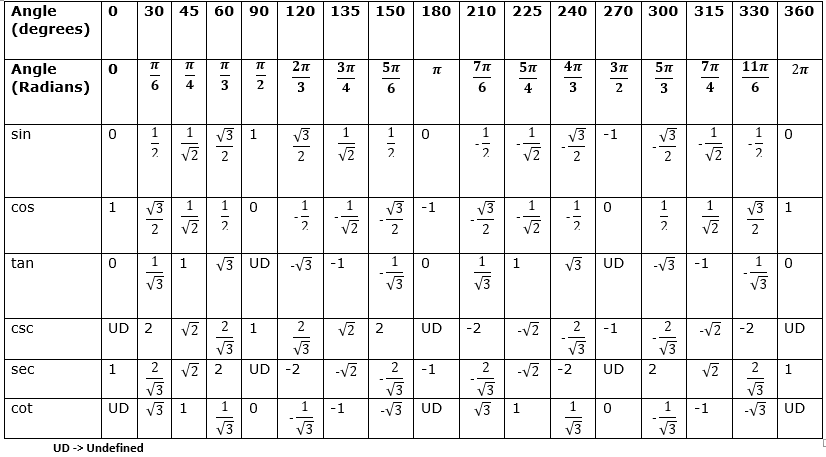
Image: cabinet.matttroy.net
Mastering the Unit Circle: A Tool for Every Situation:
The Unit Circle is not just a theoretical concept; It’s a powerful tool that finds practical applications in various disciplines:
- Trigonometry: The Unit Circle is fundamental to understanding trigonometric functions, solving trigonometric equations, and deriving identities.
- Calculus: The Unit Circle is used in calculus to find derivatives and integrals of trigonometric functions, and to understand the relationship between derivatives and rates of change.
- Physics: The Unit Circle helps to model periodic phenomena like waves, oscillations, and circular motion.
- Engineering: The Unit Circle plays a key role in designing structures, analyzing motion, and understanding electrical circuits.
Expert Insights:
Professor Maria Sanchez, renowned mathematician: “The Unit Circle is the foundation of trigonometry, providing a visual representation of angles and their corresponding trigonometric values. By mastering this concept, you’ll gain a deeper understanding of the relationships between angles and functions, unlocking a world of possibilities in mathematics and beyond.”
Dr. David Lee, esteemed physicist: “The Unit Circle is an invaluable tool in physics, allowing us to model periodic phenomena with elegant mathematical precision. It provides a framework to understand the behavior of waves, oscillations, and circular motion, underpinning many crucial physical systems.”
Actionable Tips to Master the Unit Circle:
- Visualize: Draw the Unit Circle on a piece of paper and label its quadrants, key angles, and coordinates of points. This will help you to visualize the relationships between angles and trigonometric values.
- Memorize: Start by memorizing the values of sine, cosine, and tangent for the key angles (0°, 30°, 45°, 60°, 90°). You can use mnemonic devices or flashcards to aid in memorization.
- Practice: Solve practice problems using the Unit Circle. This will help you to solidify your understanding and develop your problem-solving skills.
- Applications: Explore the applications of the Unit Circle in different areas like trigonometry, calculus, physics, and engineering. This will help you to appreciate its practical value and broaden your understanding.
Trig Values Table 0 To 360 Degrees Pdf
https://youtube.com/watch?v=Ii0YdafU8-M
Conclusion:
The Unit Circle is not just a table of values; It’s a visual representation, a key to understanding the intricate dance between angles and trigonometric functions. It opens doors to a world of possibilities, empowering you to solve complex problems and understand the world around us with greater depth. Take the first step on this journey of discovery, explore the Unit Circle, and unlock the secrets of trigonometry!






