Have you ever found yourself staring at a complex math problem involving logarithms and wondered, “How on earth do I solve this?” Don’t worry, you’re not alone. Logarithms, while fascinating in their own right, can be intimidating, especially when you have to manually calculate them. Fortunately, the advent of calculators has simplified the process of finding logarithms significantly.
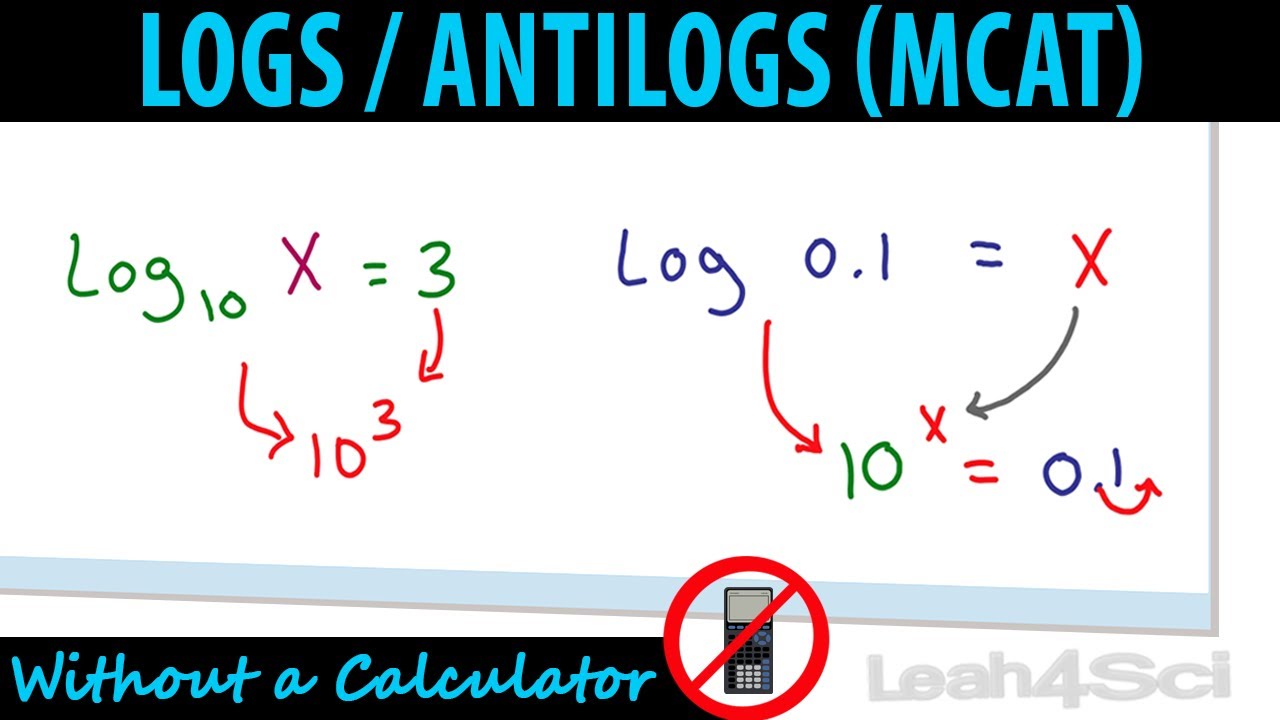
Image: www.youtube.com
In this comprehensive guide, we’ll delve into the world of logarithms, explore the different types of logarithms, and ultimately provide you with a practical roadmap on how to find logarithms using a calculator. Whether you’re a student tackling a challenging math assignment or a professional utilizing logarithms in your field, this article will equip you with the essential knowledge and skills to navigate the world of logarithms with confidence.
The Basics of Logarithms
At its core, a logarithm answers the question: “To what power must we raise a given base to get a specific number?” In simpler terms, it’s the inverse operation of exponentiation. Let’s break it down with an example:
Consider the equation 102 = 100. Here, 10 is the base, 2 is the exponent, and 100 is the result. Now, representing this equation in logarithmic form, we get log10(100) = 2. This reads as “the logarithm of 100 to the base 10 is 2.”
The “log” notation signifies that we’re dealing with logarithms. The subscript “10” indicates the base, and the number “100” inside the parentheses is the argument. The result of this logarithmic function is 2, which is the exponent that gives us 100 when the base is 10.
Types of Logarithms
While the concept of logarithms might seem straightforward, there are different types of logarithms that you’ll encounter:
- Common Logarithm (log10): This logarithm uses 10 as its base. It’s widely used in various scientific and engineering applications.
- Natural Logarithm (ln): This logarithm uses the mathematical constant *e* (approximately 2.71828) as its base and is often denoted as “ln.” It plays a crucial role in calculus and other advanced mathematical topics.
- Logarithm to any Base: While common and natural logarithms are the most common, logarithms can be defined using any positive number (except 1) as their base. This flexibility allows for various applications across different disciplines.
Using a Calculator to Find Logarithms
Most calculators are equipped with dedicated keys for finding both common logarithms (log10) and natural logarithms (ln). Here’s a step-by-step guide on how to use a calculator to calculate logarithms:

Image: www.youtube.com
Step 1: Identify the Logarithm Type
First, determine whether you need to find the common logarithm (log10) or the natural logarithm (ln) of a number. This will dictate which button you press on your calculator.
Step 2: Input the Number
Enter the number for which you want to find the logarithm into your calculator.
Step 3: Press the Logarithm Button
Press either the “log” button (for common logarithms) or the “ln” button (for natural logarithms) on your calculator.
Step 4: Read the Result
The calculator will display the logarithm of the number you entered. This is the exponent to which you must raise the base (10 for common logarithm, *e* for natural logarithm) to get the original number.
Example:
Let’s say you want to find the common logarithm of 100. Here’s how you would do it:
- Enter “100” into your calculator.
- Press the “log” button.
- The calculator will display “2,” which is the logarithm of 100 to the base 10.
Tips for Using a Calculator for Logarithms
Here are some helpful tips to enhance your logarithm calculations:
- Pay Attention to Parentheses: When using complex expressions involving logarithms, ensure proper use of parentheses to maintain the correct order of operations. For instance, “log(2 + 3)” is different from “log(2) + log(3).”
- Use Decimal Places: Calculator results often display logarithms to several decimal places. Adjust the number of decimal places to suit your specific needs and round off if necessary.
- Understand Logarithmic Properties: Familiarize yourself with the properties of logarithms, such as the product rule, quotient rule, and power rule. These can simplify complex logarithmic calculations and make them easier to solve on a calculator.
- Check Your Calculator’s Manual: If you’re unsure about any specific function or feature on your calculator, consult its manual for detailed instructions and examples.
Beyond Basic Logarithms
While a calculator simplifies the process of finding logarithms, it’s essential to understand the underlying concepts and applications of logarithms. Logarithms are used in various fields, including:
- Science and Engineering: Logarithms are crucial for expressing complex relationships and calculations in physics, chemistry, and engineering.
- Finance: Logarithms play a role in calculating compound interest and financial growth.
- Computer Science: Logarithms are used in algorithms, data structures, and complexity analysis.
- Statistics: Logarithmic transforms are often applied to data in statistical analysis to normalize distributions and improve model fitting.
How To Find Log With Calculator
Conclusion
Mastering the art of finding logarithms with a calculator empowers you to effectively tackle complex mathematical problems, understand scientific principles, and navigate various applications across different fields. This guide has equipped you with the step-by-step process and valuable tips to confidently find logarithms using a calculator. Remember to always strive for a deeper understanding of the concepts behind these calculations, as it will ultimately enhance your problem-solving capabilities and open up new avenues for exploration and discovery. As you continue your journey into the realm of logarithms, embrace the challenges, explore the fascinating applications, and unlock the power that logarithms hold.






